Menu
Substance
A summary of Penrose's summary of physics and math.
|
Define addition and multiplication maps for complex numbers:
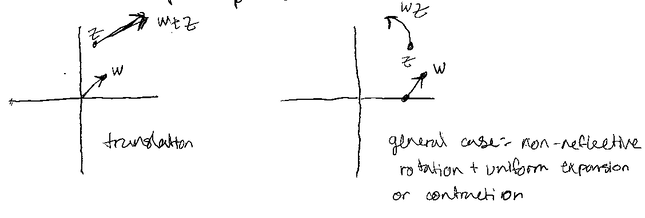
\[z \mapsto x + z\] \[z \mapsto xz\] with \(z, w \in \mathbb{C}\) On the complex plane: Roots of Unity
For a complex value \(z\), \(e^{z} = 1 + \frac{z}{1!} + \frac{z^{2}}{2!} + \frac{z^{3}}{3!} + ...\) converges for all \(z\). Suppose, \(z, w \in \mathbb{C}\) and \(z = log(w)\) with \(w\) having polar coordinates \([r, \theta]\). Then \(z = log(r) + i\theta\).
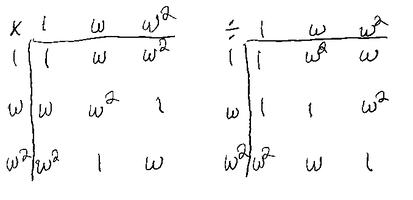
Note: above, \(log(w)\) can \( = z\) but also any \(z + 2\pi in\) where \(n = \) any positive, nontrivial number. If \(w\) has polar coordinates \(r, \theta\), note also that \(w = re^{i\theta}\). Note also, \(e^{\pi i} = 1\), \(e^{2\pi i} + 1 = 0\) (the Euler formula). The unit circle in \(\mathbb{C} = \) the circle with \(r = 1\) given by \(w = e^{i\theta}\) for real \(\theta\). \(z^{n} = w \rightarrow z = w^{\frac{1}{n}}\) when \(z = n\) and \(n =\) positive integer, we have \(n\) roots \(w^{\frac{1}{n}}\). Suppose \(w = 1\), \(log(w)\) has solutions \(0, 2\pi i, 4\pi i, 6\pi i, ...\) and we get \(1 = e^{0}, e^{2\pi i/n}, e^{4\pi i/n}, ...\) for positive values of \(1^{\frac{1}{n}}\). We can write \(\epsilon = e^{2\pi i/n}\) st. \(e^{0} = 1, e^{2\pi i/n} = \epsilon, e^{4\pi i/n} = \epsilon^{2}\), etc. These are \(n\) equally spaced points on the unit circle, called roots of unity. For a given \(n\), the \(n^{th}\) roots of unity are a finite multiplicative group (meaning closed under multiplication), the cyclic group denoted \(\mathbb{Z}_{n}\). Example, \(n = 3\) gives \(1, w, w^{2} = 1, e^{2\pi i/3}, e^{4\pi i/3}\) then \(w^{3} = 1\), \(w^{-1} = 2\) and so on:
0 Comments
Fundamental Theorem of Algebra: any polynomial of the form \(a_{0} + a_{1}z + a_{2}z^{2} + a_{3}z^{3} + ... + a_{n}z^{n} = 0\) where \(a_{i}\) are complex numbers and \(a_{n} \ne 0\) will always have a solution.
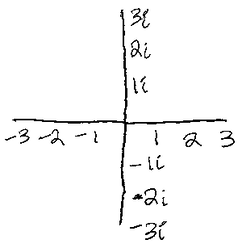
power series = infinite sum of the form \(a_{0} + a_{1}x + a_{2}x^{2} + a_{3}x^{3} + ... = \sum_{i = 0}^{\infty} a_{i}x^{i}\) the number \(z = x + iy\) is represented on the complex plane:
for any power series \(a_{0} + a_{1}z + a_{2}z^{2} + a_{3}z^{3} + ...\) where \(z\) is a complex plane value, there exists a circle of convergence centered at 0 where the series converges for all values inside the circle
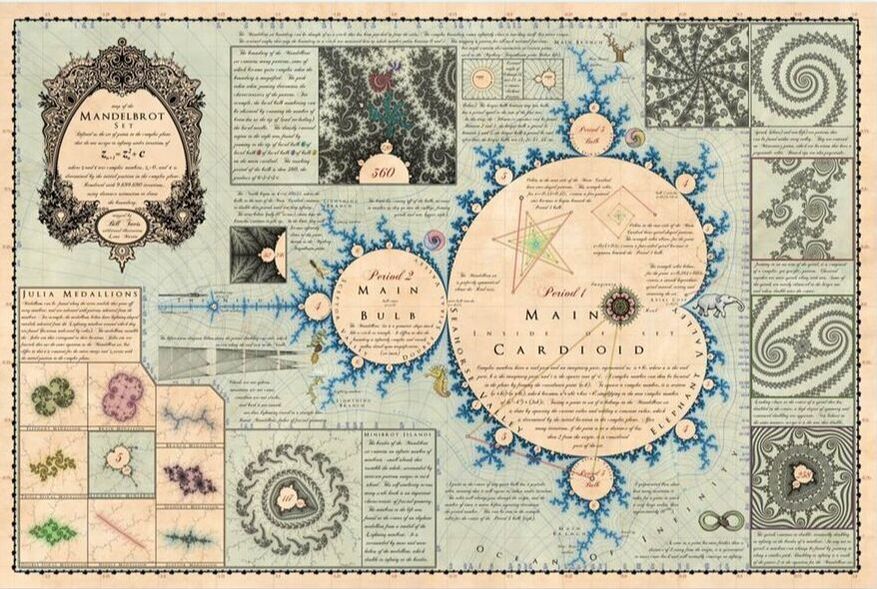
- if radius of convergence = 0, then series diverges for all \(z \ne 0\) - if radius = \(\infty\), series converges for all \(z\) The radius of convergence is determined by the singularity of the series closest to the origin in the complex plane. Poles = singularities of a complex valued power series arising from the vanishing of a polynomial in reciprocal form. The Mandelbrot Set
Let \(c = \) some point in the complex plane
Let \(z = 0\) We iteratively apply the transformation \(z \mapsto z^{2} + c\) for a given \(z\) instance, 1. if \(z\) goes to \(\infty\), then color \(c\) white 2. if \(z\) is confined to a finite region, color \(c\) black So \(z\) is just some starting point, ie., the origin and \(c\) is the location on the complex plane in consideration so a computer would do the above for each \(c\) in the plane. Condition 1 = unbounded sequence Condition 2 = bounded Mandelbrot set = the set of \(c\) values where we have a bounded sequence |
Archives
April 2022
Categories |
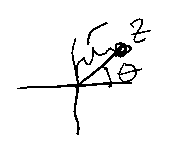
 RSS Feed
RSS Feed